Part transitions in random circuit sampling

The computational complexity of quantum methods arises from the exponential development of the Hilbert house dimension with system dimension. On near-term quantum processors whose sensible complexity is proscribed by noise, random circuit sampling (RCS) has emerged as probably the most appropriate candidate for a beyond-classical demonstration. The interaction between computational complexity and noise is highlighted by latest RCS experiments with rising system sizes and constancy^4,5,6,9,10, though classical algorithms have additionally superior considerably^{11,12,13,14,15,16}. RCS is, arguably, the entry level into the realm of classically intractable issues for any experimental quantum processing platform¹⁷. The reason being that RCS circuits will be optimized to maximise the pace of quantum correlations with iSWAP-like gates^2,18,19,20 whereas stopping potential simplifications within the corresponding classical emulations¹⁷. This intensifying quantum–classical competitors motivates two questions. Are there well-defined boundaries for the area the place the exponentially giant Hilbert house is, in truth, leveraged by a loud quantum processor? Extra importantly, can we set up an experimental observable that straight probes these boundaries?

On this work, we offer direct perception to those two questions utilizing RCS on a two-dimensional grid of superconducting qubits. We show that the interaction between quantum dynamics and noise can drive the system into distinct phases. The boundary between these phases will be resolved utilizing finite-size research with a constancy estimation approach referred to as cross-entropy benchmarking (XEB)^2,3,4,21. Reaching the specified part of maximized complexity requires a noise fee per cycle under a crucial threshold whose worth is set by the expansion fee of quantum correlations.

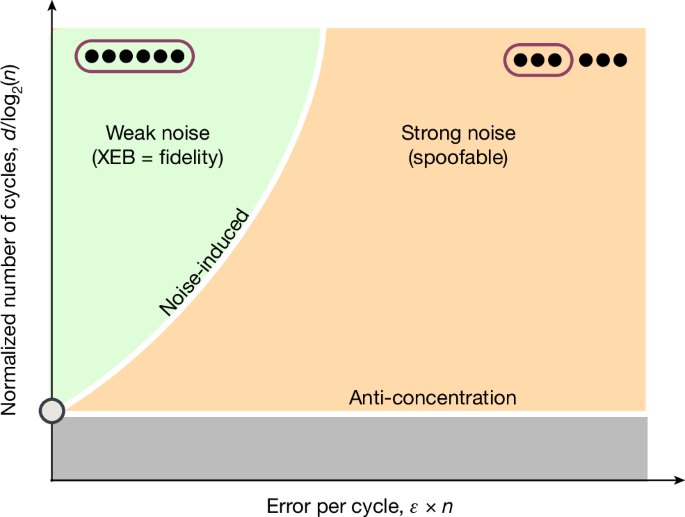
The construction of those phases is schematically illustrated in Fig. 1. Pushed by the circuit variety of cycles or depth, the system first goes by way of a dynamical part transition the place the output distribution is now not concentrated in a fraction of bit strings. Anti-concentration is a key ingredient of XEB and of mathematical arguments on the complexity of simulating noiseless RCS^{2,22,23,24,25}. However, we’ll present that this can be a needed however not ample situation for international entanglement (Supplementary Info part H), which maximizes the computational value.

One part transition goes between a concentrated output distribution of bit strings from RCS at a low variety of cycles to a broad or anti-concentrated distribution. There’s a second part transition in a loud system. A robust-enough error per cycle induces a part transition from a regime the place correlations lengthen to the total system to a regime the place the system could also be roughly represented by the product of a number of uncorrelated subsystems.

The second transition is pushed by noise, particularly the error fee per cycle ϵ × n, the place ϵ is the error per gate and n is the variety of qubits. As illustrated in Fig. 1, the behaviour of quantum correlations falls into two regimes: when the error fee per cycle is giant, the state of the system will be roughly represented by a number of uncorrelated subsystems (equation (3)). This leaves the quantum system open to spoofing by classical algorithms that symbolize solely a part of the system at a time^22,26,27. Within the regime the place the error fee per cycle is sufficiently low, correlations ultimately span the complete system, thus restoring its computational complexity, and the experiment can’t be spoofed (Supplementary Info part F). The boundary between these two phases is set by the competitors between ϵ × n and the convergence of the system to the ergodic state. Reference ²⁸ studied the identical part transition for one-dimensional and all-to-all connectivity.

We discovered that XEB is a correct observable and can be utilized to resolve the aforementioned regimes experimentally, as it’s delicate to the character of the dominant correlations. Particularly, linear XEB is measured as

the place n is the variety of qubits, ({p}_{{rm{sim}}}(s)) is the best (simulated) chance of bit string s and the typical is over experimentally noticed bit strings s. We measured XEB as a operate of the variety of cycles or depth d for various system sizes to resolve the dynamical part transition (Fig. 1). The experimental outcomes are proven in Fig. 2a,b for one- and two-dimensional methods, respectively. We discovered that XEB elevated with system dimension for small d. On this case, the system state was concentrated in a fraction of foundation states. Nonetheless, for giant d, XEB decayed exponentially and approximated the circuit constancy. At an intermediate variety of cycles, we noticed a single crossing level the place all of the measured XEB curves intersected and the place XEB was roughly impartial of the system dimension. The detailed principle introduced in Supplementary Info part E reveals that that is certainly a part transition related to the position of the boundary.

a–d, At a low variety of cycles, XEB grows with the dimensions of the system. In a noiseless system, XEB will converge to 1 with the variety of cycles. Within the presence of noise, XEB turns into an estimator of the system constancy. a,b, We experimentally noticed a dynamical part transition at a hard and fast variety of cycles between these two regimes in a single (a) and two dimensions (b). The random circuits have Haar random single-qubit gates and an iSWAP-like gate as an entangler. c,d, We experimentally probed a noise-induced part transition utilizing a weak-link mannequin (see the primary textual content), the place the weak hyperlink is utilized each 12 cycles (discrete gate set; see principal textual content). c, The 2 totally different regimes. Within the weak-noise regime, XEB converges to the constancy, whereas within the strong-noise regime, XEB stays greater than predicted by the digital error mannequin. d, We induced errors to scan the transition from one regime to the opposite.

Having recognized the minimal variety of cycles at which XEB approximated the system constancy, we formulated an experimental protocol for finding the transition between the strong- and weak-noise regimes (Fig. 1). A conceptually easy set-up that highlights the underlying physics for this transition is the so-called weak-link mannequin, the place two subsystems of dimension n/2 are coupled by way of an entangling gate utilized each T cycles. Within the restrict the place T = ∞ (no weak hyperlink is utilized), the subsystems have been uncoupled and the general system converged to a product state ρ_A ⊗ ρ_B, the place ρ_A and ρ_B are the pure ergodic states of every subsystem. Including noise, we assumed the so-called depolarizing channel noise mannequin for the density matrix of every subsystem A and B (ref. ²⁹): F^d/2ρ_A/B + (1 − F^d/2)I_A/B/2^n/2, the place I_A (I_B) is the id matrix and F = e^−ϵn is the constancy. Direct substitution of this density matrix into equation (1) provides XEB = e^−ϵnd + 2e^−ϵnd/2. We use XEB = 1 for ρ_A/B and XEB = 0 for I_A/B/2^n/2.

At finite but giant T, every subsystem approached the ergodic state in lower than T cycles. The applying of a two-qubit gate between the 2 subsystems constructed international correlations, which, subsequently, decreased the XEB time period proportional to F^d/2 with some fee λ. This fee λ relies on the gate and was λ = 1/4 for the iSWAP-like gates employed in our experiment (Supplementary Info part D). Subsequently, a simplified mannequin for linear XEB is

We justify this equation by formally averaging circuit cases over two-replicas, which leads to the so-called inhabitants dynamics formalism (Supplementary Info part D). We probed this behaviour by measuring XEB experimentally as a operate of d, as proven in Fig. 2c. We employed a noise-injection protocol that successfully modified the gate fidelities in our quantum circuits (Supplementary Info part C2) and present outcomes comparable to totally different noise ranges. We used the discrete set of single-qubit gates chosen randomly from Z^pX^1/2Z^−p with p ∈ {−1, −3/4, −1/2, …, 3/4} and Z and X the corresponding Pauli matrices. We noticed that within the weak-noise regime, XEB converged to the anticipated constancy of the complete system, F^d. This was as a result of F was sufficiently excessive such that F^d dominated the contribution to XEB. Alternatively, XEB was considerably above F^d within the strong-noise regime owing to the dominant contribution of twoλ^d/TF^d/2 to XEB. These outcomes exemplify the competitors between the exponential decay of worldwide correlations proportional to ϵn and the entangling fee between subsystems (proportional to 1/T on this instance).

The transition between the 2 totally different noise-induced phases was extra clearly seen by fixing d to some values and ranging the efficient noise degree (F). We noticed that XEB displays a non-trivial scaling distinct from F^d (Fig. Second). Specifically, the speed of decay with respect to errors decreased at greater error charges. That is additionally in line with 2λ^d/TF^d/2 being dominant at excessive errors.

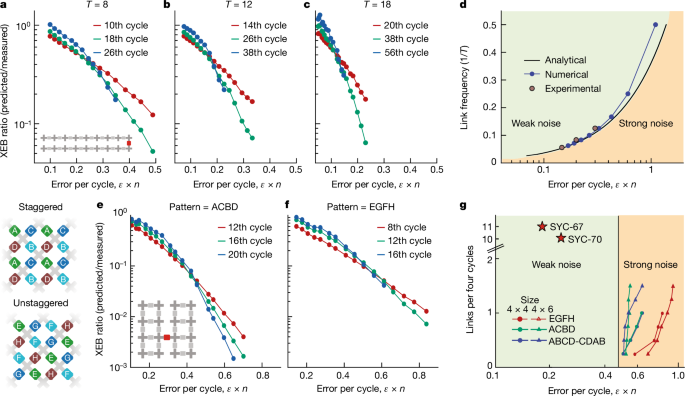
To experimentally find the crucial worth of the error per cycle (or equivalently, F) the place the dynamical exponent of XEB modified, we outlined a modified order parameter F^d/XEB, which is asymptotic to a definite worth of 1 (0) within the weak (sturdy) noise regime. The transition between the 2 limits turned a discontinuity when d → ∞, indicating a part transition for finite ϵn ≈ κ_c, the place the crucial worth κ_c could be a operate of λ and T. Within the transition area, we noticed the finite-size crucial behaviour the place F^d/XEB was roughly a operate of (ϵn − κ_c)d. This was revealed within the order parameter for various numbers of cycles d crossing at a single level, as will be verified from equation (2) and numerically for the circuits used within the experiment. The experimentally obtained F^d/XEB, proven in Fig. 3a–c,e,f, certainly manifested the anticipated crucial behaviour. For ϵn ≲ κ_c, the order parameter elevated as d was elevated, whereas for ϵn ≳ κ_c, the order parameter decreased as d was elevated. On the crucial level ϵn ≈ κ_c, the datasets crossed and the order parameter was roughly impartial of d. We attribute the slight drift within the crossing level between totally different depths to potential systematic errors within the experimental estimation of F.

a–c, Experimental noise-induced part transitions as a operate of the error per cycle for a number of intervals T of the weak-link mannequin (discrete gate set) (T = 8 (a), T = 12 (b) and T = 18 (c)). As T will increase, the crucial worth of noise or error per cycle turns into decrease. d, Part diagram of the transition with analytical, numerical and experimental knowledge. The experimental knowledge have been extracted from the crossing of the biggest variety of cycles scanned. e,f, Experimental transitions in two dimensions with totally different patterns: staggered ACBD (e) and unstaggered EGFH (f; discrete gate set). g, Numerical part diagram of the two-dimensional part transition. We present the crucial level for various sizes and patterns. The qubits are organized as proven within the inset of e. For fastened dimension, we elevated the variety of bridges (such because the crimson coupler in e) till all bridges have been utilized (4 and 6 for the 4 × 4 and 4 × 6 methods, respectively), denoted as hyperlinks per 4 cycles in g. For all of the patterns, we delimited a decrease sure on the crucial error fee of 0.47 errors per cycle to separate the area of sturdy noise the place XEB did not characterize the underlying constancy and the place international correlations have been subdominant. The experimental outcomes proven in Fig. 4 (SYC-67) and in Supplementary Info part C1 (SYC-70) are represented by crimson stars, that are effectively throughout the weak-noise regime.

We extracted the crucial noise fee for various hyperlink frequencies 1/T experimentally, numerically and analytically (Fig. 3d). When evaluating with the practical type ({epsilon }nsimeq 4/Tlog 2) predicted by the analytical weak-link mannequin, we noticed considerable deviations when the hyperlink frequency approached 1/2, which corresponds to the common one-dimensional chain. The deviation occurred as a result of the weak-link mannequin was now not relevant on this regime. A extra correct description is offered by a generalization of this mannequin in Supplementary Info part E1.

To elucidate the character of the noise-induced part transition for 2 and extra dimensions, we prolonged the weak-link mannequin from two subsystems to a variety of subsystems equal to the variety of qubits (see Supplementary Info part E2 for particulars). For depths d ≫ 1, the subsystems converge both to an applicable ergodic state or to a state proportional to the id matrix, as within the weak-link mannequin. Within the noiseless case, there are two stationary configurations, with both all subsystems within the id matrix or all within the ergodic state. We expanded the noisy state round these two configurations utilizing the so-called dilute flipped spin growth. This generalizes equation (2) to

the place the primary (second) time period on the right-hand facet corresponds to the growth across the id matrix (ergodic state). See Supplementary Info part D for the derivation. The index okay describes the overall variety of subsystems not equal to the corresponding international state and okay! is its factorial. The primary time period on the right-hand facet corresponds to the low-weight Pauli paths approximation in ref. ²⁷. Lastly, λ = 1/4 for the iSWAP gate, as within the weak-link mannequin.

The noise-induced part transition seems within the thermodynamical restrict n → ∞ at fastened ϵn and (d/log n) (see Supplementary Info part E for particulars). We discovered

The second issue on the right-hand facet corresponds to the worldwide constancy and dominates within the weak-noise regime ({epsilon }nll {rm{ln}},4). The primary issue describes the convergence to anti-concentration (Supplementary Info part E), in accordance with refs. ^22,23, and dominates within the strong-noise regime ({epsilon }ngg {rm{ln}},4). On this case, the primary time period on the right-hand facet of equation (3) prevails, and by taking small okay, the state will be roughly represented by a number of uncorrelated subsystems. On the one hand, as captured by equation (3), this transition corresponds to qualitatively totally different configurations. Alternatively, each regimes correspond to an ordered part. The noise-induced part transition is pushed by a management parameter (analogous to a magnetic subject in an Ising mannequin) that scales with the system dimension (variety of qubits). That is loosely like Fréedericksz transitions in liquid crystals³⁰ and is qualitatively totally different from the quantum to classical transition mentioned in ref. ³¹. The transition mentioned here’s a competitors between the finite fee of convergence to the general ergodic state and the constancy per cycle. The transition in ref. ³¹ is a contest between native interactions and the error fee per qubit and is expounded to the error threshold of quantum error correction codes.

The 2-dimensional experimental outcomes are proven in Fig. 3e,f for a 4 × 4 sq. grid of qubits and two totally different circuit buildings, whereby the two-qubit gates have been utilized both in a staggered (Fig. 3e) or an unstaggered (Fig. 3f) vogue. As in a single dimension, the 16-qubit system was divided into two halves related by a single iSWAP-like gate utilized each 4 cycles. For each circuit buildings, we noticed the same crossing between F^d/XEB measured at three totally different cycles, with a better worth of ϵn noticed for the unstaggered patterns.

We numerically evaluated crucial noise charges for methods of various sizes and circuit buildings with out weak hyperlinks, together with each the staggered and unstaggered patterns and the ABCD-CDAB sample utilized in ref. ⁴. Right here, we used Haar random single-qubit gates (Supplementary Info part D).

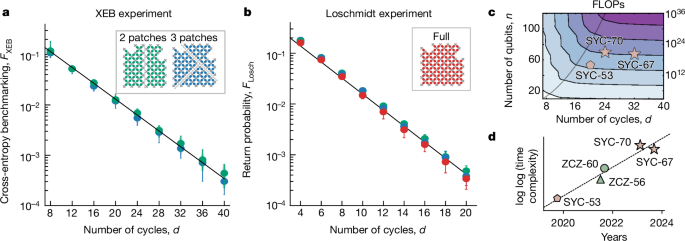
The vertical line in Fig. 3g provides a decrease sure for the noise-induced part transition. Moreover, the noise-induced part transition for the discrete gate set used within the experiment occurred at greater noise charges (Supplementary Info part F2). We in contrast this decrease sure with the 67-qubit and 70-qubit RCS experiments that we are going to current subsequent. We obtained the error per cycle by becoming the exponential decay of the constancy (Fig. 4). It’s evident that these experiments fell effectively throughout the weak-noise regime, satisfying the requirement to totally make the most of the computational capability of the noisy quantum processors.

a, Verification of RCS constancy with logarithmic XEB. The complete system is split into two (inexperienced) or three (blue) patches to estimate the XEB constancy for a modest computational value. We used the discrete gate set of single-qubit gates chosen randomly from Z^pX^1/2Z^−p with p ∈ {−1, −3/4, −1/2, …, 3/4}. For every variety of cycles, 20 circuit cases have been sampled with 100,000 pictures every. The stable traces point out the XEB estimated from the digital error mannequin. b, Verification of RCS constancy with Loschmidt echo. The inversion was executed by reversing the circuit and inserting single-qubit gates. On this case, the Loschmidt echo variety of cycles doubled. c, Time complexity estimated as a operate of the variety of qubits and the variety of cycles for a set of circuits. As a working definition of time complexity, we used the variety of FLOPs wanted to compute the chance of a single bit string underneath no reminiscence constraints. The stable line signifies the depth at which correlations unfold to the total system and the FLOPs with depth go from exponential to polynomial. d, Evolution of the time complexity of the RCS experiments. The dashed line represents doubly exponential development as a information for the attention.

We demonstrated beyond-classical RCS by performing an experiment on a 67-qubit Sycamore chip (Fig. 4). These random circuits adopted the identical two-dimensional ABCD-CDAB sample as ref. ⁴. The only-qubit gates have been chosen randomly from the discrete set Z^pX^1/2Z^−p with p ∈ {−1, −3/4, −1/2, …, 3/4}. We present in Supplementary Info part B the constancy of the elementary operations of the random circuit. On common, we achieved a read-out error of 1.3(0.5) × 10⁻², a dressed two-qubit Pauli error fee of 5.5(1.3) × 10⁻³ that may be additional decomposed right into a single-qubit Pauli error fee of 1.0(0.5) × 10⁻³, and an intrinsic two-qubit simultaneous error of three.5(1.4) × 10⁻³. An intrinsic Pauli error fee of three.5 × 10⁻³ corresponds to a median constancy of 99.72%. We validated the digital error mannequin by taking a look at patched variations of the random circuit (inset in Fig. 4a), the place slices of two-qubit gates have been eliminated to create patched circuits for which every patch XEB will be verified for a modest computational value. The full constancy was then the product of the patch fidelities. Computing XEB over full circuits is, at current, an intractable classical job. We, thus, estimated the constancy after 32 cycles utilizing the discrete error mannequin, acquiring 0.1%. This elevated depth was doable due to the considerably decreased errors in contrast with earlier processors. We collected over 70 million pattern bit strings for a single circuit at this depth. In Supplementary Info part SC1, we report fidelities for an additional XEB experiment, SYC-70, executed on 70 qubits and with 24 cycles. In Fig. 4b, we confirm these extracted fidelities with the Loschmidt echo, the place we use the identical circuit and its inversion to return to the preliminary state. We noticed good settlement with the XEB experiment and the digital error mannequin.

Lastly, we estimated the equal classical computational value of RCS with the tensor community contraction technique^{7,11,12,13,14,15,16,18,32,33,34}. With this technique, ref. ¹⁵ classically sampled the RCS experiment carried out in 2019⁴ in 15 h utilizing 512 GPUs. Reference ¹⁶ additionally carried out this job with the same value. Moreover, one other group computed⁷ the corresponding XEB, which confirmed the predictions of ref. ⁴, which is a tougher computational job than noisy sampling. Therefore, these notable enhancements in classical algorithms considerably raised the beyond-classical threshold. For completeness, in Supplementary Info part H, we additionally research matrix product states, a well-liked tensor community variational illustration of one-dimensional quantum states with restricted entanglement^35,36,37. We discovered that given present supercomputer reminiscence constraints, matrix product states failed to achieve the experimental constancy and provided worse efficiency than tensor community contraction.

We report enhancements in tensor community contraction methods that resulted in decrease estimated computational prices for simulated RCS (Supplementary Info part G). Determine 4c reveals the time complexity or variety of floating-point operations (FLOPs) (the variety of actual multiplications and additions) as a operate of the variety of qubits and cycles required to compute a single amplitude on the output of a random circuit with out reminiscence constraints, as we’re optimizing solely the contraction ordering of the underlying tensor community. The time complexity on this context is rigorously outlined because the minimal contraction value achievable when it comes to FLOPs per amplitude¹², which we approximated by optimizing the contraction ordering. This served as a proxy decrease sure for the hardness of each sampling and verification. For a hard and fast variety of qubits and rising variety of cycles, there was a notional crossover within the scaling of the time complexity from exponential to linear. Given a loud experimental set-up, this suggests an optimum variety of cycles for the trade-off between computational time complexity and constancy. Past the crossover, constancy decreases sooner than the time complexity will increase. The crossover variety of cycles is in line with a scaling (sqrt{n}). A line following the practical type (d=Asqrt{n}), the place n is the variety of qubits, has been added as a information to the attention. Word that that is associated to the variety of cycles at which ‘typical’ entanglement is achieved (Supplementary Info part H) and is a stronger requirement than the anti-concentration of the output distribution. For each 67 and 70 qubits, 24 cycles was deep sufficient to saturate the exponential development of this time complexity. Determine 4d reveals the expansion of the time complexity with out reminiscence constraints of the biggest RCS experiments run over the previous couple of years.

A sensible estimate of the computational assets to simulate RCS must have in mind the finite FLOPS (FLOPs per second) supply of a supercomputer in addition to its reminiscence constraints and different limitations reminiscent of finite bandwidth. Desk 1 reveals estimates of the runtime for the approximate simulation of the biggest cases of RCS from refs. ^4,5,6 and the present work when utilizing the state-of-the-art strategies mentioned in Supplementary Info part G. For these estimates, we thought-about sampling 1 million uncorrelated bit strings at a constancy like that of the experiment utilizing the present top-performing supercomputer, Frontier, which performs 1.7 × 10¹⁸ single-precision FLOPS of theoretical peak efficiency unfold throughout GPUs with 128 GB of RAM every. This required the computation of 10 million approximate chance amplitudes of uncorrelated bit strings for use in rejection sampling¹⁸. Regardless of notable progress in reaching tensor contraction algorithms which can be embarrassingly parallelizable over every GPU^14,32, we discovered that this system broke down for the a lot deeper SYC-67 circuits with 32 cycles given the tight reminiscence constraints. In consequence, an estimated decrease sure for the sampling value turned considerably extra prohibitive. Assuming a distributed use of all RAM and underneath the unrealistic assumption of negligible bandwidth constraints, the computational value was round 1 × 10⁴ years (Desk 1). Within the untested case during which we expanded working reminiscence to all secondary storage and nonetheless ignored the bandwidth, we obtained an estimate of 12 years.

In conclusion, our experiment offers direct insights on how quantum dynamics interacts with noise. The noticed part boundaries lay out quantitative steerage to the regimes the place noisy quantum units can correctly leverage their computational energy. As well as, we current new RCS outcomes with an estimated constancy of 1.5 × 10⁻³ at 67 qubits and 32 cycles or 880 entanglement gates, comparable to greater than doubling the circuit quantity in comparison with ref. ⁴ for a similar constancy. International correlations dominate XEB within the weak-noise part, which protects RCS in opposition to ‘spoofing’ assaults, in distinction to boson sampling³⁸, the place all identified metrics for latest experiments^39,40,41 are dominated by native correlations⁴². Trying ahead, regardless of the success of RCS in quantifying the obtainable coherent assets, discovering sensible functions for near-term noisy quantum processors nonetheless stays an impressive problem. Licensed randomness technology^43,44 might be a promising candidate for such an software (Supplementary Info part I).